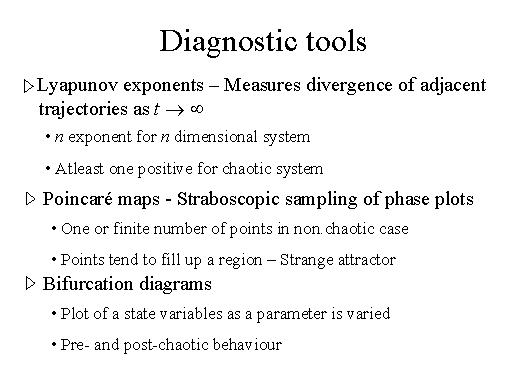
There are several ways to diagnose chaos. I mention here very briefly two of them. One is called the Lyapunov exponent, which is a measure of how the adjacent trajectories diverge as time tends to infinity. For an n dimensional system, there are n Lyapunov exponents, and it has been known in chaos literature that at least one of the exponents has to be positive. So, we will try to determine what are the Lyapunov exponents for these sets of equations when we try to simulate and see under what conditions any of them is positive. There is also something else called a Poincaré map, which is nothing but a stroboscopic sampling of the phase plots. If you have a chaotic system, you will have a large number of points. So, the points tend to fill up a region. It is also sometimes called the strange attractor. However, for a periodic system or a non-chaotic system there are only a finite number of points. So, we will use these two tools to see whether our system is chaotic or not.

---