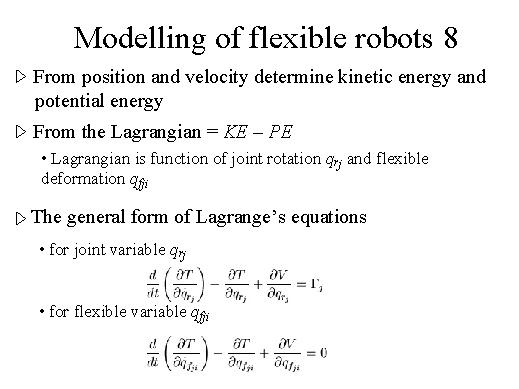
The way to do that is we use what is called as the Lagrangian formulation. In the Lagrangian formulation, for each position vector we take the derivative of the position vector which gives us the velocity. The velocity is next multiplied by mass to give the kinetic energy and then that gives us the term which is T which is the kinetic energy term. We also need to find what is called as the potential energy. In the case of flexible manipulators the potential energy comes from gravity, the weight effect and also the fact that some energy is stored in the bending vibration of the beam itself. So, we can find the Lagrangian and this formula here tells us how to take the derivative of each terms of this Lagrangian to find the so called equation of motion.
The first equation of motion has to do with the rigid variables, the arm itself is moving as a rigid variable and the second set of equations of motion has to do with the flexible variables are the bending portion of the equation of motion. Both of these together can be written in a compact matrix form which is given in this last line, last equation. It says the following: we can conclude the following from this equation of motion: that the rigid portion is mrr into qr. plus this is a term which represents the Coriolis and the centripetal term. This is a term which represents the gravity term. This is equal to the external torque which is applied by the motor. This is what we would expect for a rigid manipulator. But it turns out for a flexible manipulator that there is also a coupling term between the rigid variables and the flexible variables by means of this. Likewise, for the flexible equation, the flexible terms qf are also related to the rigid term by this coupling mrf. And the other key point to notice in this equation is that there are no external forces for the flexible term. This is the set of equations which we can derive once we know the transformations and once we can find the kinetic and potential energy.