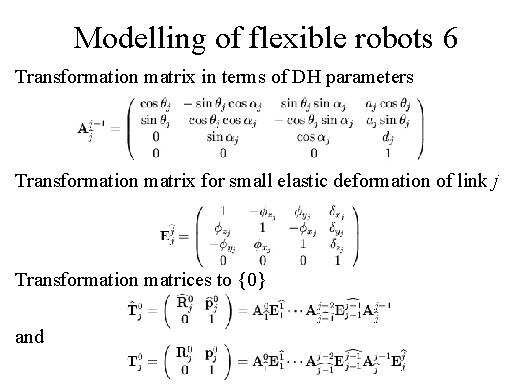
It turns out that the rigid transformation can be written in this form which consists of some cosine of certain angles and the Denavit-Hartenberg parameters a and b and a. The flexible transformation, with the assumption that it is small, can be written in this form where the diagonal elements are 1. Basically what we are assuming is the cosine of the angle is 1 and sine of the angle is the angle itself. The transformation from fixed coordinate system the base to the last link, the end-effector, is a multiplication of transformation matrices, which corresponds to rigid, flexible, rigid, flexible all the way to the end transformation.

---