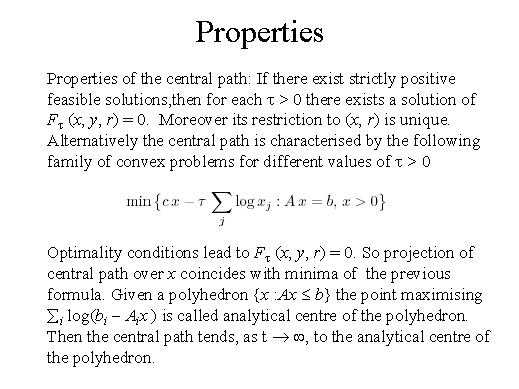
If there exist strictly positive feasible solutions, then for each t > 0 there exists a solution of
Ft (x, y, r) = 0.
Moreover its restriction to (x, r) is unique.
Alternatively the central path is characterised by the following family of convex problems for different values of t > 0.
Optimality conditions lead to Ft (x, y, r) = 0. So projection of central path over x coincides with minima of the previous formula. Given a polyhedron {x :Ax £ b} the point maximising åi log(bi - Aix ) is called analytical centre of the polyhedron.
Then the central path tends, as t ® ¥, to the analytical centre of the polyhedron.