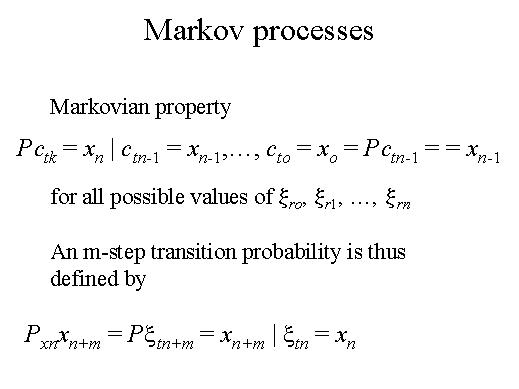
The occurrence of a future state in a Markov process depends on the immediately preceding state and only on it. If t0 < t1 < …tn (n = 0, 1, 2, …) represents points in time, the family of random variables ctk is a Markov process if it possesses the following Markovian property for all possible values x_ro, x_r1, …, x_rn.
The probability P_n-1x_n = Pc_tk = x_n½x_tn-1 = x_n-1 is called the transition probability. It represents the conditional probability of the system being in x_n at t_n, given it was in x_n-1 at t_n-1. This probability is also referred to as the one-step transition because it describes the system between t_n-1 and t_n.
An m-step transition probability isthus defined as follows.