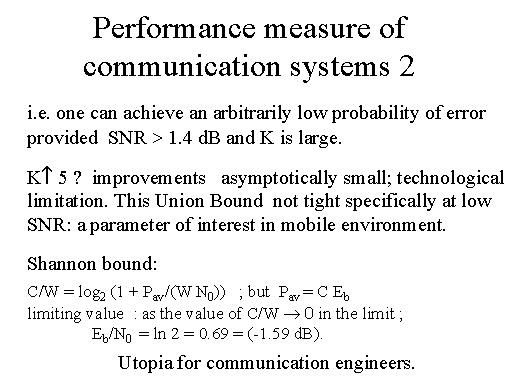
If we have E_b/N₀ not greater than 1,4 dB, one could have low probability of error by making K as large as possible, but then one has limitation.
We start with the expression on Shannon bound where C/W is given to you as log to 1 + P average divided by noise power. But then, if we do that, and if you take the value of CW tending to 0 in the limit, one gets the value of E_b/N₀ as -1,59 dB. That means basically what Shannon indicated in his famous paper in 1948 and 1949 is that what one could have a small probability of error at as low a value of E_b/N₀ as equals to -1,59 dB.
But then Shannon's expression of Shannon's theorem is only existential, it does not tell you how you can achieve this. Therefore this particular bound of 1,59 dB is considered to be utopia for communication engineers.