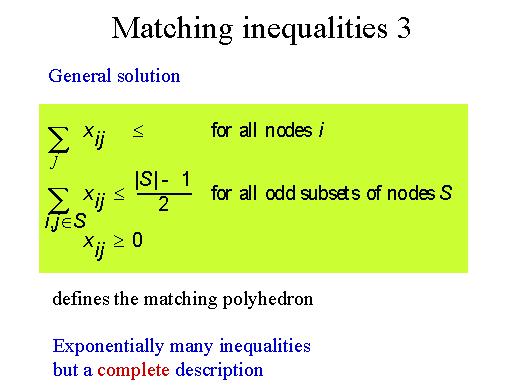
If we generalise this result we should consider all possible odd subsets S of nodes. And for each one of them we impose that not more than (S-1)/2 arcs can belong to a matching. So we have this inequality. In total we have the degree constraints and the odd subset constraints. Question: do they define the matching polyhedron? Answer: yes, they do. We have exponential number of inequalities, but a complete description and this is a very strong result. Again we have a strong result in connection with a polynomial problem. And the theory says that we cannot expect such a nice behaviour for problems which are NP-complete.