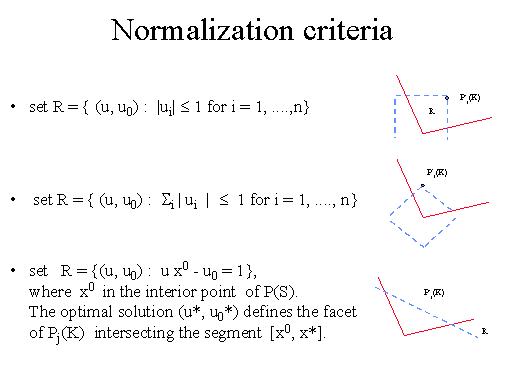
There are different criteria by which the set R may be defined. For instance we may choose R as the set of the vector whose either infinity norm or 1 norm is not greater than 1.
Alternatively, we can normalize the feasible vectors using the knowledge of a point x⁰ in the interior of P(S) and requiring that ux⁰-u⁰ = 1. In this last case by solving the separation problem we identify the facet of P_j(K) intersecting the segment connecting x⁰ and x* [x⁰, x*].
If one knows a vector x⁰ interior to P(S) then can use the normalization ux-u⁰ = 1.
This choice corresponds to choosing the facet that separates x* from x⁰ that intersect the segment [x⁰, x*].
Alternatively one can use the infinite norm requiring that the absolute value of each component of 1 is less than 1. This choice introduce vertex in the cone that do not belong to the extremal rays and so the corresponding solution may be not facet inducing.
An other choice is to use norm 1 ecc. In any case the separation problem may be solved by finding the optimal solution of a different linear programming problem with 3n variables an constraints.