Well, let's now see how to solve the separation problem with respect
these families of inequalities.
Assume that x* is the optimal solution of a given linear relaxation K
of the mixed 0-1 problem and that the j-th component of x* is fractional.
Then there exist at least one facet inducing inequality o Pj(K)
that is violated by x*.
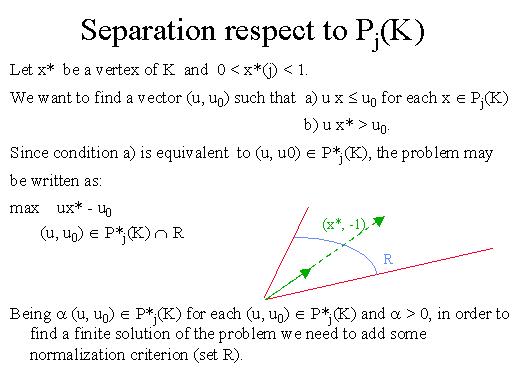
In particular, we may look for the valid inequality of the form ux -u0
which is maximally violated by x*.
This task may be accomplished simply by maximizing the quantity ux*- u0,
that is the violation in x*, on the polyhedral cone P*j(K),
that is to say by solving a suitable linear programming problem.
Note that, since the cone P*j(K) is
unbounded, in order to obtain a finite optimal solution we must introduce
some normalization criterion, represented by the set R.