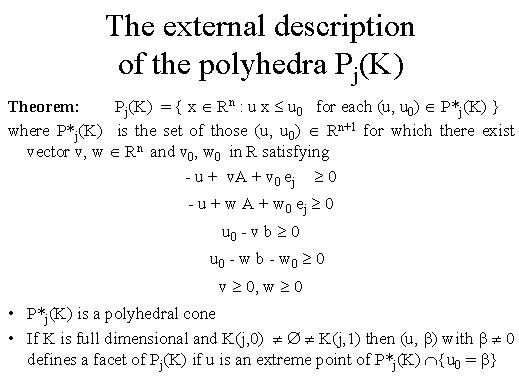
This aim can be accomplisched by using the tecniques that allow to obtain the linear description of projections of polyhedra onto given subsets of variables. As a final result one obtains that the vectors (u, u0) which define valid inequalities for the polyhedron P_j(K) are those belonging to a particualr cone P*_j(K). By definition this cone is the projection onto the (u, u0) variables of the polyhedron defined by this system of constraints. For our purposes, what is important to note here, is that a valid inequality for P_j(K) may be obtained simply by solving a linear programming problem of roughly twice the size of the original problem and taking the (u, u0) components. Moreover, it can be proved that under general hypotheses, each point belonging to an edge of the polyhedral cone p*j identifies a facet of the polyhedron P_j(K).