Let's now consider as a second example, a general cutting plane method
for mixed 0-1 integer linear programming based on a convexification approach.
This method derives from a more general theoretical framework developed
by Balas and others authors and has connections with both disjuntive programming
and lift and project methods.
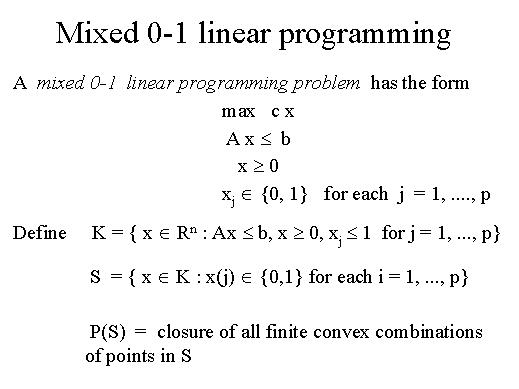
In a mixed 0-1 linear problem besides the usual linear constraints we
impose the condition that some of the variables, let's say the first p,
have to assume values in the set 0, 1. In the following K will stand for
the feasible set of the linear relaxation, S will stand for the set of
the feasible solutions of the mixed problem and P(S) for the closure of
the convex hull of S.