Fortunately, if we are interested in finding an optimal solution on S,
or for optimization purposes, we do not need a complete linear description
of P(S) but all we need to do is represent this polyhedron in the neighborhood
of an optimal solution. In other words, we may solve the integer problem
as soon as we are able to produce a relaxation Q of P(S), that is to say
,a polyhedron containing P(S), with the property that at least one of
the optimal solutions of the integer problem is an optimal solution of
the linear problem on Q.
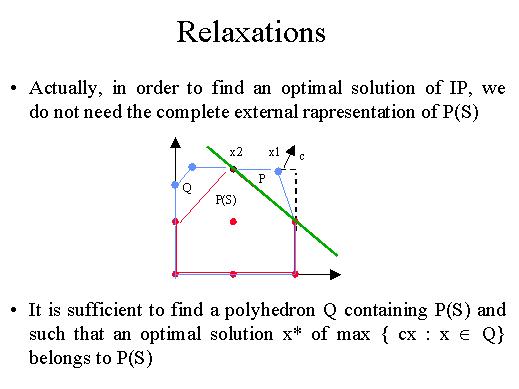
As an example consider the same figure as in the previous slide. If we
add to P the constraint corresponding to the green line we obtain a relaxation
Q which contains P(S) properly since Q contains two blue vertices of P.
Nevertheless, if we optimize the linear function cx on the polyhedron
Q we get as an optimal solution the point x2 that belongs to S.
How are we to obtain such a relaxation? The idea is to iteratively construct
it by adding new constraints to the initial formulation P of the problem
in such a way that no feasible solution is lost and possibly large parts
of P not contained in P(S) are cut off.