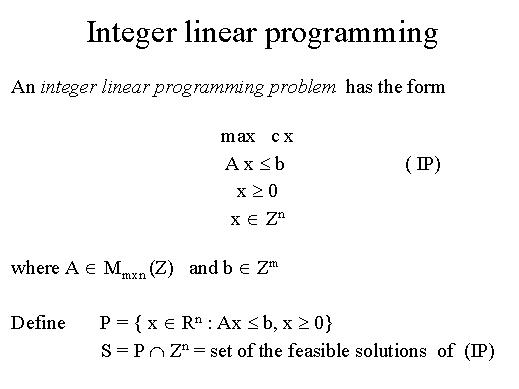
An integer linear programming problem is that of optimizing (let say
maximizing ) a linear function cx over the set of the solutions of a finite
number of linear inequalities and under the condition that the components
of the solution have to take only integer values. In other words the set
S of the feasible solutions of the problem is the intersection of the
polyhedron P defined by the linear constraints of the problem and the
integer lattice formed by the elements of Zn.
We may assume that all the data that define the instance are integer numbers.