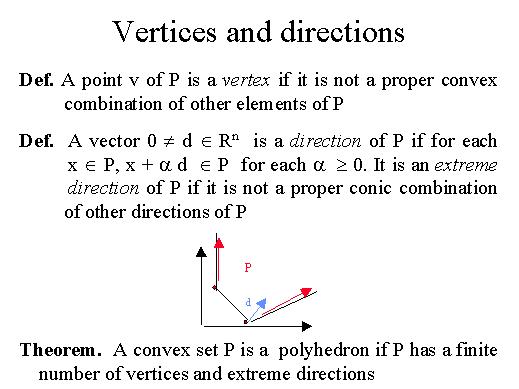
Polyhedra are particular convex sets. Given a polyhedron P, we say that
an element v of P is a vertex of P if it cannot be written as a convex
combination of other elements in P.
We also say that a vector d of Rn is a direction of the polyhedron P if
for each element x of P the ray pointed in x and having direction d is
entirely contained in P. Moreover we say that d is an extreme direction
of P if d cannot be written as a conic combinations of other directions
in P. For example, represented in the figure is an unbounded polyhedron
with two vertices corresponding to the two red points and two extreme
directions represented by the red arrows. We can obtain a different characterization
of polyhedra by saying that a polyhedron is any convex set having a finite
number of vertices and extreme directions.