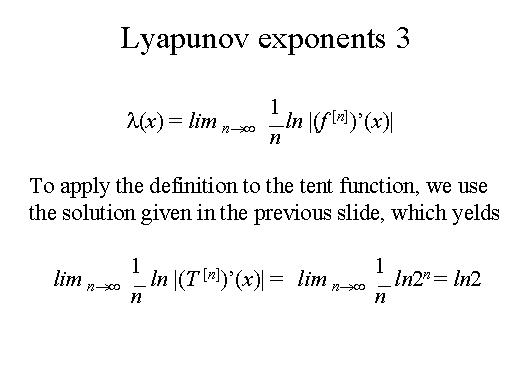
Let J be a bounded interval, and f : J ® J continuously differentiable on J. Fix x in J, and let l(x) be defined by the following, provided that the limit exists. In that case l(x) is the Lyapunov exponent of f at x. If l(x) is independent of x wherever l(x) is defined, then the common value of l(x) is denoted by l, and is the Lyapunov exponent of f. The definition honours the 20th century Russian mathematician A.M. Lyapunov.

---