While formally the Lagrange equations of motion are sufficient for the solution of the problem, in the case of motion with one point fixed it is often convenient to use a different set, known as Euler’s equations of motion.

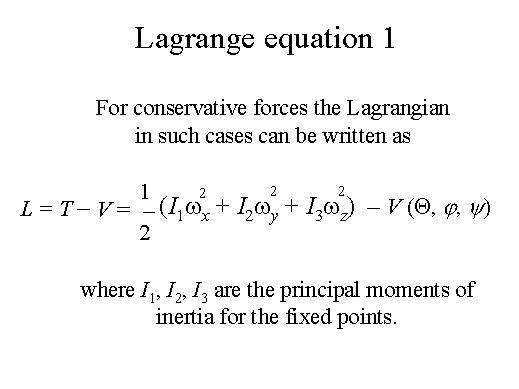
For conservative forces the Lagrangian in such cases can be written as follows, where I₁, I₂, I₃ are the principal moments of inertia for the fixed points. The components of the angular velocity along the principal axes, w_x, w_y, w_z, can be expressed in terms of the Euler angles which relate the principal axis system to some space set of axes. By this means the entire Lagrangian can be written as a function of the three rotation angles Q, f, y. It will be remembered that the generalised force corresponding to a generalised coordinate of rotation is the component of the impressed torque along the axis of rotation.