If the maximum operating speed is above the first critical speed the rotor has to be considered "flexible". When a rotor is not rigid it is not possible to substitute to the actual unbalance or eccentricity distribution their resultant force and moment. Theoretically we need a balancing plane for each elementary rotor element. Practically several balancing planes are foreseen for longer rotors (generally from 5 to 7). The eccentricity distribution is obviously unknown, and cannot be measured, so generally we measure the effect of the eccentricity, which are the vibrations in correspondence of the bearings. 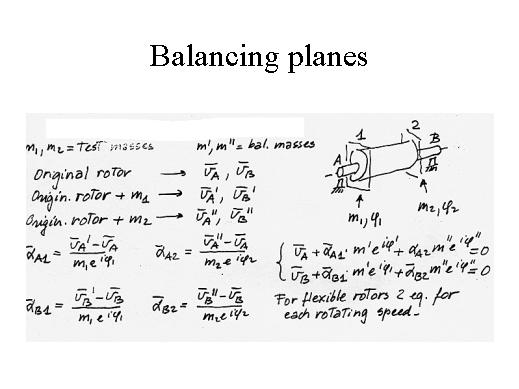A general balancing procedure can be used which is the influence coefficient method. It consists in measuring (generally on the bearings) the vibration vectors at the different speeds, in the original condition of the rotor, and with a single trial mass subsequently on each balancing plane. For each speed a complex influence coefficient can be calculated for each balancing plane and for each measuring point, by calculating the vector difference of the vibration due to the original unbalance and the single trial mass minus the vibration due to the original unbalance alone, divided by the trial mass. This represents the effect of an unit mass. If we suppose linearity of the system (which is not always true), we calculate the vibration on a bearing due to a certain mass on a certain balancing plane by multiplying the corresponding influence coefficient by the mass (which is represented by a complex number or a vector having an intensity and a phase angle). We can therefore impose null vibration on both bearings, for each rotating speed, and calculate the balancing masses. For rigid rotors we have 2 equations for the 2 bearings (only one speed). For flexible rotors we have 2 equations for the 2 bearings for each speed. If the balancing planes are less than twice the interested speeds, than a least square procedure may be used for minimising the vibrations instead of making them null.

---